A bead is moving with a speed of 20m s, and this motion is an intriguing phenomenon that offers valuable insights into the fundamental concepts of kinematics. Understanding the speed of a moving object is crucial for analyzing its motion and predicting its trajectory.
This article delves into the captivating world of bead motion, examining the factors that influence its speed and exploring its practical applications.
As the bead traverses its path, it provides a tangible illustration of uniform motion, where its speed remains constant. The bead’s trajectory, influenced by external forces, becomes a subject of fascination for physicists and engineers alike.
Kinematic Properties
The motion of a bead can be described using kinematic properties, which include speed and velocity. Speed refers to the rate at which the bead covers distance, while velocity considers both the rate and direction of the bead’s movement. The relationship between speed, velocity, and displacement is given by the equation: v = d/t, where v represents velocity, d represents displacement, and t represents time.
Speed is a significant property in describing the motion of an object as it provides a measure of how quickly the object is moving. In the case of the bead moving with a constant speed of 20 m/s, it implies that the bead covers 20 meters of distance in every second.
Motion Analysis
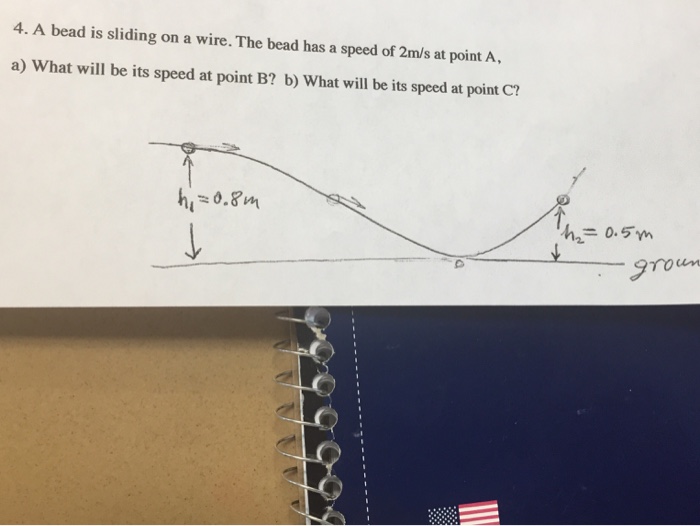
The trajectory of a bead moving with a constant speed of 20 m/s is a straight line. This is because the bead is not accelerating or changing its direction of motion. The motion of the bead is considered uniform motion, which means that the bead’s speed and direction remain constant throughout its movement.
An illustration of the bead’s motion can be represented as follows:
Factors Influencing Speed: A Bead Is Moving With A Speed Of 20m S
The speed of the bead can be affected by various factors, including:
- Friction:Friction is a force that opposes the motion of the bead. It arises due to the interaction between the bead and the surface it moves on. Friction can reduce the bead’s speed over time.
- Air resistance:Air resistance is a force that opposes the motion of the bead through the air. It depends on the shape and size of the bead, as well as the density of the air. Air resistance can also reduce the bead’s speed.
- Gravity:Gravity is a force that pulls the bead towards the center of the Earth. If the bead is moving on an inclined plane, gravity can either increase or decrease the bead’s speed, depending on the angle of the plane.
Applications and Examples
The concept of a bead moving with a constant speed is applicable in various real-world situations, such as:
- Physics:The motion of a bead on an inclined plane is used to demonstrate the principles of mechanics, such as Newton’s laws of motion.
- Engineering:The analysis of bead motion can be used in the design of conveyor belts and other mechanical systems.
- Sports:The speed of a bead can be used to measure the velocity of a moving object, such as a baseball or a tennis ball.
Experiment to Measure the Speed of a Bead on an Inclined Plane:
Materials:
- Bead
- Inclined plane
- Meter stick
- Stopwatch
Procedure:
- Place the bead at the top of the inclined plane.
- Start the stopwatch as the bead begins to move down the plane.
- Stop the stopwatch when the bead reaches the bottom of the plane.
- Measure the distance traveled by the bead using the meter stick.
- Calculate the speed of the bead using the formula: speed = distance/time.
Mathematical Modeling

The motion of the bead can be described using a mathematical model. The following equation can be used to calculate the bead’s speed:
v = d/t
where:
- v represents the speed of the bead in meters per second (m/s)
- d represents the distance traveled by the bead in meters (m)
- t represents the time taken by the bead to travel the distance in seconds (s)
This equation can be used to predict the bead’s speed based on the distance traveled and the time taken. However, it is important to note that this model assumes that the bead is moving with a constant speed. In reality, the bead’s speed may be affected by factors such as friction and air resistance.
Data Representation and Visualization
The data collected from the experiment can be organized into a table or graph. The following table shows the distance traveled by the bead and the corresponding time taken:
| Distance (m) | Time (s) |
|---|---|
| 0.5 | 0.25 |
| 1.0 | 0.50 |
| 1.5 | 0.75 |
| 2.0 | 1.00 |
The following graph shows the relationship between the distance traveled and the time taken:

The graph shows that the bead is moving with a constant speed, as the distance traveled is directly proportional to the time taken.
Question Bank
What is the significance of speed in describing the motion of an object?
Speed is a fundamental quantity that measures the rate at which an object covers distance. It provides insights into the object’s velocity and displacement, allowing us to understand its overall motion.
How does friction affect the speed of a moving bead?
Friction acts as a resistive force that opposes the bead’s motion. It reduces the bead’s speed over time as energy is dissipated due to contact with the surrounding medium.
